Fractions, elements, and capabilities.
A big portion of my profession up to now has been spent musing over the right way to engineer classroom environments that infuse which means into these three mathematical constructions. On the subject of polynomial factoring, the realm mannequin has offered probably the most success. After connecting 2-digit by 2-digit multiplication, the realm mannequin turns into a stupendous visible to make sense of the “provides to ___; multiplies to ___” phrase that echoes across the room.
However we don’t hold the realm mannequin round ceaselessly. As soon as we’ve used the mannequin to construct which means, we mobilize that understanding in additional symbolic conditions in a cautious, deliberate march towards mathematical abstraction.
On the subject of designing these types of symbolic, pattern-based duties, I see them entering into three potential instructions:
- Here’s a polynomial, what are the elements? (Enter each single textbook ever written)
- Listed here are some parameters, construct a polynomial that satisfies them. (Construct a trinomial that has a non-1 GCF and in addition an element of “x – 3” and so on.)
- Meet within the center. (Listed here are a set of each polynomials and elements, match them up).
After some experimentation, I found that the “meet within the center” supplies a curious risk when working with polynomial factoring: College students should choose elements and phrases based mostly on the factoring patterns we’re aiming to solidify, but additionally should regulate what’s /not/ being chosen. That’s, with each selection towards finishing all of the matches, the strain is placed on to go away items that work nicely collectively. Due to this, the sense-making amplifies as you go alongside. This seems like a dream situation for the trainer.
The Process
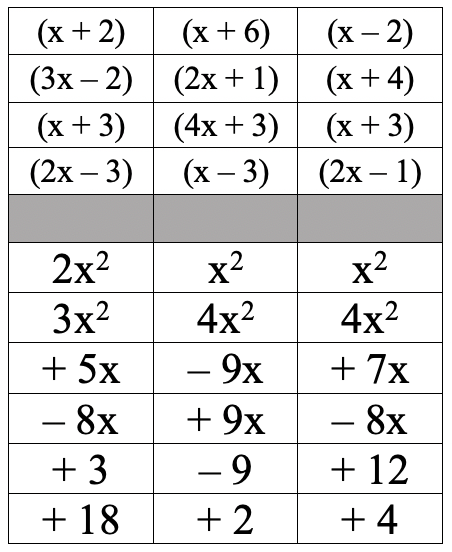
I constructed six trinomials for this specific matching activity. There was a contact of intentional design in my decisions, however nothing extremely advanced. Basically, I used to be attempting to create some good beginning locations, but additionally embody sufficient attainable overlap to end in quite a lot of methods. (After all, you may add, adapt, or subtract items to your coronary heart’s content material). I printed off the polynomials on a single piece of cardstock and handed out scissors to every group.
As soon as all of the items had been reduce out, the scholars had been requested to match (and multiply) two elements from the highest part with three phrases from the underside part in an effort to reconstruct six true trinomial multiplication statements. Every of the polynomials should be utilized in precisely one assertion.
I additionally had a single instance ready to make clear the method. It didn’t contain any of the elements from this checklist of six, however confirmed how the elements are chosen and multiplied and the phrases from the underside part are mixed to type trinomials.
As the coed teams labored, I discovered the next educating prompts useful:
- What helps you slim down the choices for pairings?
- Are there any elements that can’t be paired?
- Which numbers provide the most data?
- How may you create that “4x2” time period?
- How does the addition/subtraction within the elements affect your decisions?
We did this primary set as a bunch exercise shortly after using the realm mannequin to know the symbolic sample. Scholar teams then constructed their very own set of six polynomials to commerce with a neighbouring group. It was very enjoyable to hearken to their rationale as they tried to construct an attention-grabbing set.
Finally, this can be a comparatively easy concept that units the stage for mathematical pattern-seeking and sense-making, and that’s a candy spot I’m in search of in my classroom.
NatBanting
P.S. In opposition to my higher judgement, the decision to the duty may be discovered right here, however (possibly) it is best to spend a while matching earlier than ruining it for your self.